Building Math Metacognition in Three Easy Steps

We know that students who have a gap between doing math and thinking like a mathematician will struggle with mathematics. And as students move through the grades, if we don’t do something to fill in the gap, it will just keep getting bigger and bigger.

“For students to become more metacognitive, they must be taught the concept and its language explicitly….Metacognition is not generic, but instead is most effective when it is adapted to reflect the specific learning contexts of a specific topic, course, or discipline.” (Chick, 2017)

The research shows that these cognitive and metacognitive skills are interconnected and interdependent. That’s why the mathematics standards were intentionally designed with two types: Standards for Mathematical Content and Standards for Mathematical Practice. The standards brought together a vast array of research to clearly state the importance of simultaneously developing conceptual understanding, computational fluency, and problem-solving skills. But what the standards were not clear on is how to integrate the two types and what the mathematical practices look like as students progress from grade to grade.

So, how do we better integrate these practices that in turn support metacognition and ensure we are meeting students’ developmental needs? There are three steps we must take.

1) Value the Two Types of Standards

First, we need to understand the value of both types of standards. By articulating both content and practice, the standards define both what students should know and be able to do in mathematics and how they should be thinking and speaking about mathematics.

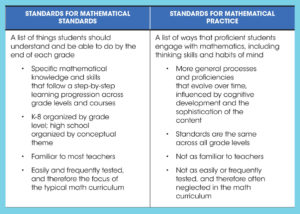
As teachers, it is important that we deeply understand the standards themselves, their issues, and the processes involved—to think about the thinking—and thereby help students develop the necessary conceptual understandings as well as the procedural and metacognitive skills required to be successful with math. That’s what the learning progressions support, which leads us to the second step…

2) Determine the Progressions of the Mathematical Practices

Second, we need to clearly understand the grade-level expectations of each of the Standards for Mathematical Practice.

The eight Standards for Mathematical Practice have focus. They are the same for all grade levels. They have rigor. They represent ways that students can engage with and think about mathematics. But they lack coherence—the careful, deliberate, and progressive development of ideas based on how students’ mathematical knowledge, skill, and understanding develop over time.

Although they have been narrowed to a manageable number and each one has a description of processes and proficiencies that mathematically proficient students should engage in, the descriptions are not clear or specific enough to provide concrete guidance on how they develop over time or how to support them from grade to grade.

So, we need to look at the Learning Progressions of the grade-level expectations for each Practice. For example,

With each Standard for Mathematical Practice and its description serving as the ultimate goal, the grade-level expectations provide a clear and cumulative path to mathematical metacognition.

So, is that all we need? No, but it’s a beginning.

3) Teach the Skills within the Practices

Third, we need to use step-by-step processes to teach the skills within each practice standard and then to model and guide students to master the practices. For example, here are the processes for Practice 1.

Then, it is imperative that we support our students to think like mathematicians by asking questions that foster metacognition and encourage them to speak like mathematicians when making ownership statements that demonstrate that they are actively integrating both cognitive and metacognitive skills, both mathematical content and mathematical practice.

Your task is to support students to use these practices on a daily basis. Your task is to drive this thinking and this speaking through direct conversations like these…

First Grade

TEACHER: What is the problem asking you to do?

STUDENT: The problem is asking me to find twelve minus five.

TEACHER: What are some other words you can use to explain the problem?

STUDENT: This is the same as taking five things away from a group of twelve. When I take away my answer will be smaller.

TEACHER:  How do you want to solve it?

STUDENT: I can draw a group of twelve circles, X out five, and count how many are left.

TEACHER:  What can you do if you get stuck?

STUDENT: If I get stuck, I can use counters instead of my drawing. The answer is seven, but I can check my work by adding five plus seven and make sure it equals twelve. Then I know I have the answer.

TEACHER:  How did making sense of the problem and persevering in solving it help you?

STUDENT:  I have to think about what the problem is. Then I can try different ways to solve it until I see the answer is right.

Sixth Grade

TEACHER:  What is the problem asking you to do?

STUDENT:  The problem is asking to create a ratio of loaves between two different types breads.

TEACHER:  How could you go about solving the problem?

STUDENT:  I think I need to look at the number of loaves of wheats to the rye bread and compare them.

TEACHER:  What is the most efficient way to solve the problem?

STUDENT:   I can set up the comparison by writing the ratio of 5 loaves of wheat bread to 2 loaves of rye bread in math form.

TEACHER:  Does this make sense?

STUDENT:  When I compare my work to my partner’s, she got the same ratio, so I know this makes sense.

TEACHER:  How did making sense of the problem and persevering in solving it help you?

STUDENT:  It helped me to understand what the problem is really asking me to do. Then, I could figure out how to solve it and then to work on it until it was finished. 

High School Algebra I

TEACHER: What is the problem asking you to do?

STUDENT: The problem is asking me to find the slope of a line using two sets of points.

TEACHER: What information are you given in the problem?

STUDENT: I was given the two ordered pairs which are four, two and three, sixteen.

TEACHER: How will you use those ordered pairs to find the slope of the line?

STUDENT: I start by reminding myself that in an ordered pair, the first number represents x and the second represents y. I also remember that slope is defined as rise over run and that rise over run is change in y divided by change in x which also called m. This forms the formula for slope. I substitute the two sets of points given in the problem into that formula and I can solve the problem.

TEACHER: What other strategies might you try?

STUDENT: I can solve it another way by graphing, which I’ve done before. Understanding various approaches to solutions is important because it helps me make better sense of what I’m doing and helps me to persevere if I struggle.

TEACHER: How did making sense of the problem and persevering in solving it help you?

STUDENT: It helped me to understand what the problem is really asking me to do. Then, I could figure out how to solve it and work on it until it was finished.

These are just a few examples of “what students are thinking and saying” as they demonstrate application of the practices. As these examples show, students are learning the language of mathematics and the grade-level math content at the same time they are learning the metacognitive skills of examining and applying their own reasoning.

Can you imagine having mathematical conversations like these with your students? When you are integrating the practices into your daily math instruction, you will.