Teaching Kids to Speak Like Mathematicians

Because mathematics uses a lot of symbols to convey information (+, -, x, =), it is easy to assume all students can understand what is being taught because math is a “universal language.” However, the symbols represent specific language (add, subtract, multiply, equals) that is used by mathematicians. The belief of this universal language assumes that students, therefore, do not need language support. But this belief will lead educators astray.

The Common Core State Standards for Mathematics brought together this vast array of research and instructional expertise to present a clear framework for metacognition in mathematics by placing a strong emphasis on the equal importance of procedural skill and mathematical understanding. Changed the game from simply teaching students how to do math. Now it is our privilege and responsibility for teachers to ensure that students how to think like a mathematician and how to articulate their thinking and mathematical understanding—in other words, how to speak like a mathematician.

The Role of Language in Mathematics

Contrary to popular assumption, language plays a critical role not just in reading and writing, but in learning mathematics as well. Kristina Robertson suggests that we tend to think of math as not requiring a strong command of language when, in fact, “mathematical reasoning and problem solving are closely linked to language and rely upon a firm understanding of basic math vocabulary.” Therefore, as a teacher, you’re challenged with both making math lessons comprehensible and ensuring that students have the language to understand instruction and express their grasp of math concepts orally and in writing.

The Role of the Student

The student’s role is to be able to articulate their thinking. For greater academic success, students must be able to state what they are learning and why, can explain how they learn best, can articulate when they are learning and when they are struggling, and understand their role in the learning.

We call these articulations, Ownership Statements. These statements help the student articulate their thinking—their metacognition—out loud. Over time students should be able to use these statements to clarify their conceptual understanding, computational fluency, and problem-solving skills. And in doing so, they are expected to use specialized vocabulary in mathematics when articulating their understanding of problems, when solving problems, and when sharing their thinking process. This math talk also helps teachers and students recognize what students understand and when and where they are struggling. Discussing how they are thinking about math is also a way of redirecting the lesson from teacher-to-student to student-to-student.

Examples of Ownership in Action

First Grade Student

The problem says ten plus eight equals blank. I can use math words to describe what I’m going to do. The plus sign means I’m going to add ten to eight. So, I’m doing addition.

Fourth Grade Student

I notice that the denominators and numerators of the two fractions are different. It’s hard to compare fractions when the denominators are different. So, I will create a picture that represents the fractions so I can see their sizes. I can draw a pizza divided into 15 equal parts and a drawing of another pizza divided into five equal parts. I will shade in six slices in the 15-slice pizza. I will label that as 6/15. Then I will shade in three slices in the 5-slice pizza. I will label that as 3/5. I can see they are equivalent fractions.

Seventh Grade Student

I can use mathematical language to describe how I’m going to solve this problem and explain my thinking as I go. Writing an equivalent expression means rewriting it without changing its value. To solve the problem, I needed to use order of operations and the distributive property. Then, when I combined all the like terms, I had written an equivalent expression.

High School Student

The problem is asking me to determine how much Max’s account will have after five years assuming he makes no deposits or withdrawals. I know that one of the mathematical terms that apply in this situation, even though it isn’t mentioned in the problem, is “compound interest.” It is important that I label quantities appropriately because it affects my ability to solve the problem accurately. I know this is a compound interest problem so I can use a formula to help me show the meaning of the quantities. Since interest is paid semiannually, I need to divide the interest rate by two. Also, the interest is paid twice per year. It would make sense for me to find a compound interest formula. Then, I can use my calculator to compute the amount in the account after five years to the nearest cent.

The Role of the Teacher

The teacher’s role is to ask questions that get kids thinking like mathematicians and then as needed model how and/or support students to speak like mathematicians. They need to model how to articulate thinking using specialized vocabulary in mathematics. Denise Jarrett points out while most students “can be relatively proficient in social language, they must be explicitly taught to use academic language.”

Most students need a great deal of explicit instruction, modeling, and coaching before they develop the kind of speaking skills that allow them to take ownership of their learning. This makes the role of the teacher a crucial one in helping all students develop the use of academic and content language in mathematics.

Two of the most effective methods to develop the use of articulate mathematical language is to:

1. Strategically teach the appropriate use of academic and content language.
2. Ask specific questions and offer sentence frames that push students’ speaking skills so that they can articulate precise thinking and processing.

Academic Language that Fosters Metacognition

Many students struggle with academic language because their exposure to it outside of the school environment does not include advanced words and phrases. Even more absent is the opportunity to manipulate and use specific content language needed to precisely communicate mathematical thinking.

In mathematics, students make sense of problems by seeing structure and repeated reasoning and then making connections. While the use of standard English is acceptable in the social context, it is crucial that students learn the more precise language of mathematics when discussing math.

Whenever possible, model the academic language of mathematics. If students use the more common terms, restate their words as a mathematician.

Sentence Frames that Foster Metacognition

Sentence frames provide students with prompts for their speaking and thinking. They also facilitate student discussion and empower learners who may need additional support by providing an opening into the conversation with the appropriate language structure for responding to questions. Sentence frames also provide for open-ended responses that allow students to expand on their mathematical thinking and develop their reasoning ability.

Whenever possible, use these questions and frames to support students’ academic language development in both listening and speaking.

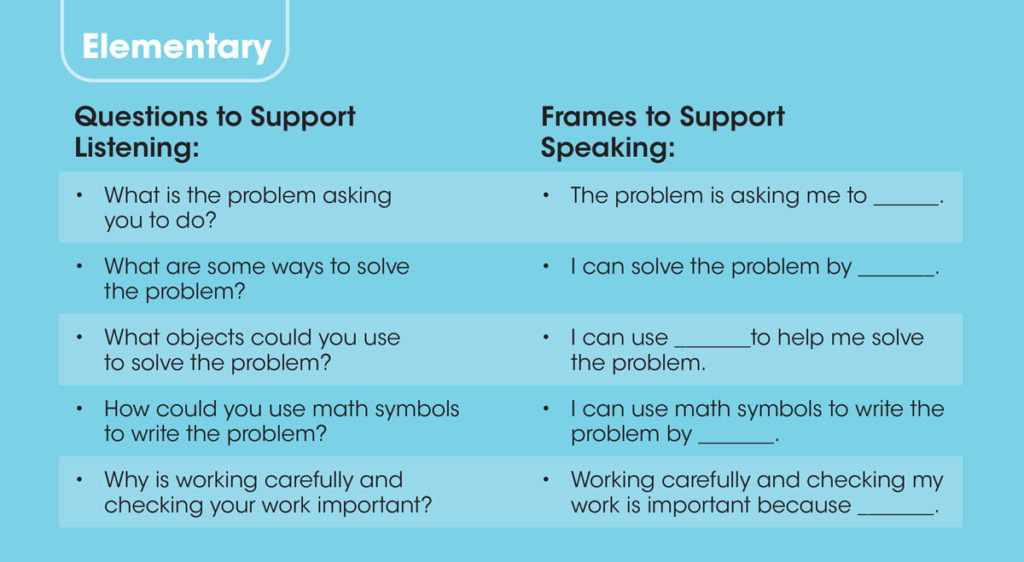
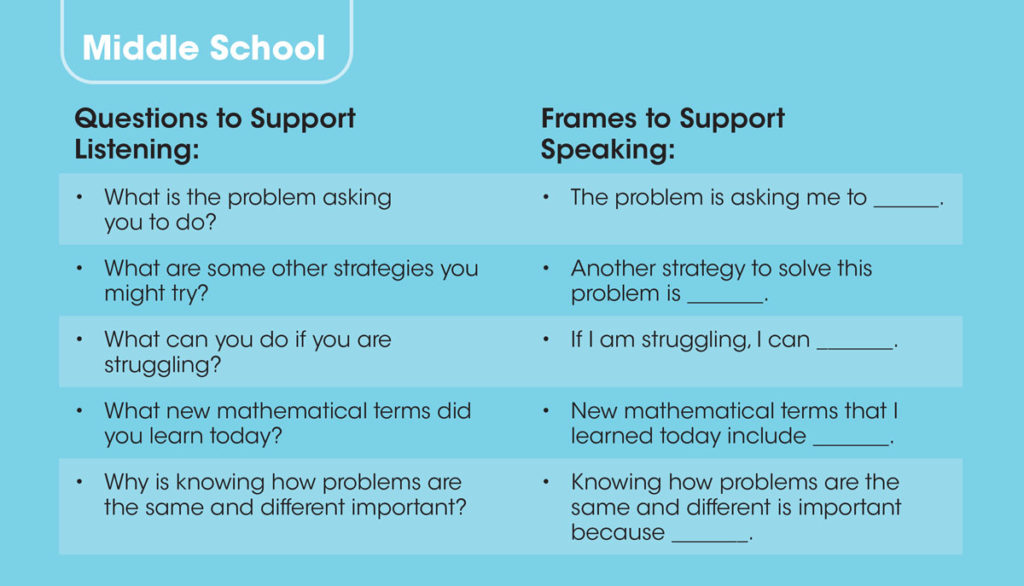
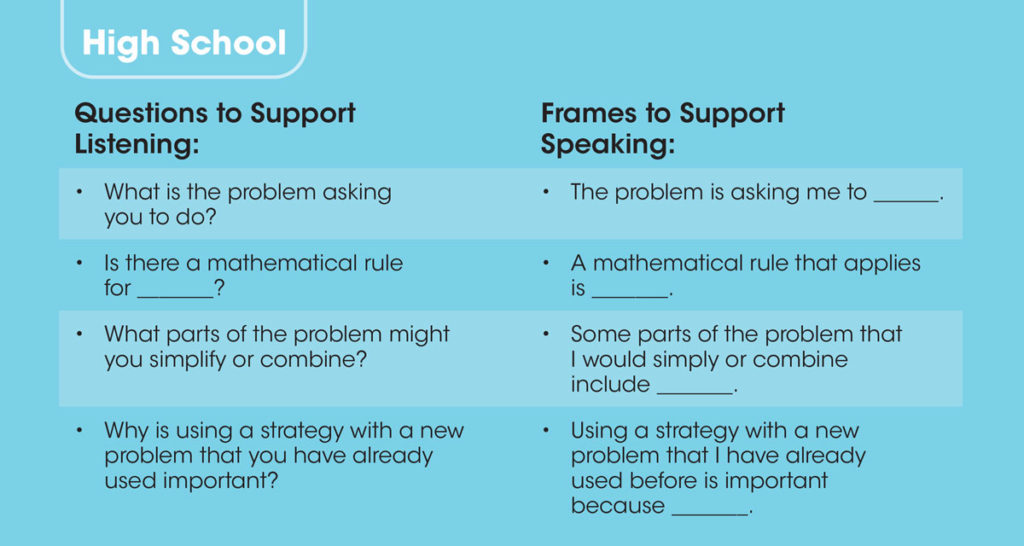
Remember, it’s the teacher’s role is to show students how to speak like a mathematician. In other words, it is important to recognize the need for a teacher to teach and model how to use academic and content language in the mathematics. Ultimately, when students begin using the vocabulary of mathematics and speaking like a mathematician, they will own their learning.