Teach Your Students to Attend to Precision

In today’s world students who are truly successful in mathematics can not only solve problems, but can explain:

• what each problem is asking,
• how they will solve the problem,
• why their solution plan makes sense, and
• how they know their answer is correct.

In other words, students must be able to think metacognitively about each and every problem and explain their thinking.

The Standards for Mathematical Practice present a helpful framework for this complex and important work. That’s why we’ve provided you with a metacognitive study of each of the mathematical practices—what this thinking looks like at each grade level, a step-by-step approach to teaching each practice, and a reflection guide to support students as they “think about their thinking.”

This article is focused on…

STANDARD FOR MATHEMATICAL PRACTICE 6:
Attend to precision.

What This Thinking Looks Like at Each Grade Level

Because the 8 Standards for Mathematical Practice are the same for all grade levels, they are not specific enough to provide much practical guidance for classroom implementation. So, we’ve provided the standard and its learning progression for every grade from Pre-K through High School to better understand how the thinking builds in tandem with students’ cognitive development and the demands of mathematical content, as shown in the example below.

A Step-By-Step Approach to Teaching Practice 6

Fostering metacognition requires a balance of explicit instruction, teacher modeling, student-centered exploration, and responsive coaching that helps students first learn the kinds of questions and thought processes they can apply, and then grow to use them on their own. These metacognitive skills come naturally to some students but not to others. Teachers must play an active role in teaching them and helping students own their mathematical learning.

Use this process to teach, model, and guide students in mastering Practice 6.

Process to attend to precision

1. 1. Explain the problem using specific mathematical language for words and symbols.
   2. Explain the problem using specific units of measure.
   3. Explain how you will solve the problem using specific mathematical language and units of measure.
   4. As you are solving the problem, explain what you are doing using specific mathematical language and units of measure.
   5. Explain your thinking in both writing and speaking.

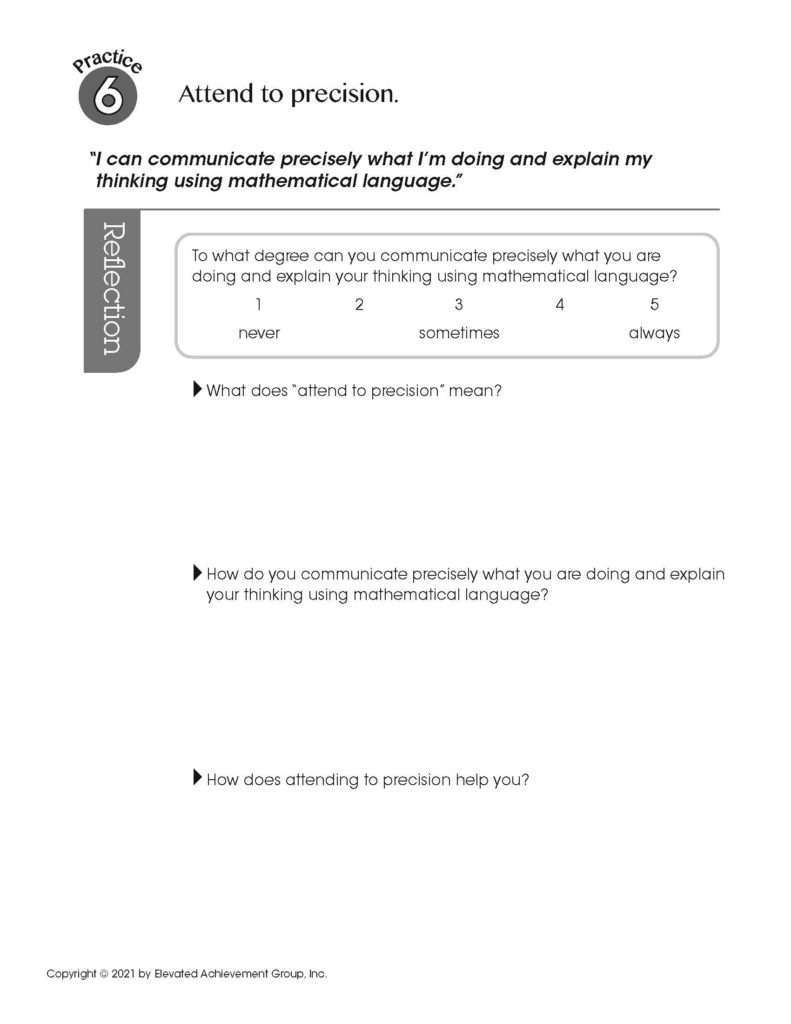
A Reflection Guide to Support Students as They “Think About Their Thinking”

As we said before, mathematics is much more than just solving the problems and checking to see if the answer is correct. It also involves each student’s understanding of what they are learning, how they can learn it, and what they have learned about their mathematical thinking along the way.

When you’re focusing on Practice 6, teach your students this routine and then encourage them to use it for each and every math problem they solve. In doing so, you will support them in thinking about, reflecting on, and sharing their thoughts as the develop ownership of their mathematics.

Foster Learnership in Mathematics

Owning their mathematics won’t be automatic. On the contrary—most students need a great deal of explicit instruction, modeling, and coaching before they develop the metacognitive habits that allow them to take ownership of their learning. The more students are able to understand their own learning process and articulate it, the easier it will be for them to improve their conceptual understanding, procedural skill and fluency, and applications. In other words, students will own their mathematics with metacognition and develop learnership.

Click here to download a resource with the processes and reflection for the Standard for Mathematical Practice 6: Attend to precision. Click below to access the same great resources for the other Standards for Mathematical Practices.

• Make Sense of Problems and Persevere in Solving Them
• Reason Abstractly and Quantitatively
• Construct Viable Arguments and Critique the Reasoning of Others
• Model with Mathematics
• Use Appropriate Tools Strategically
• Look for and Make Use of Structure
• Look for and Express Regularity in Repeated Reasoning

We hope you check out our resources…use them…share them…continue your learnership.